Hyoung Keun Kwon (MSc Data Science, 2024)
Administrative Divisions Residential Districts, and Tax Systems
Regions where administrative divisions overlap are exposed to different economic, social, and political issues than those without. While local governments in the regions compete to attract resources to satiate residents’ needs, they run into unexpected results and inefficiencies.

Recent “New Town” Projects in Korea, mainly designed to disperse the population from downtown of large cities and reduce unnecessary administrative burdens, are often located in regions where different administrative districts are intertwined, leading to many inconveniences. A prime example would be Wirye New Town (henceforth, Wirye) and its Light Rail Transit (LRT) project connecting Wirye and Sinsa, which already has two subway lines. With the national government, Seoul Metropolitan City, Songpa-Gu, Gyeonggi Province, Hanam City, and Seongnam City involved with different interests, the project has yet to start construction when it was originally planned to start ten years ago.
A common solution to such issues brought up in the media is an integration of administrative divisions. However, simply integrating the administrative districts will not solve the problem. Will the Gyeonggi, Hanam, or Seongnam governments agree with annexing Wirye to Songpa-Gu of Seoul with the consequence of losing their tax base, or vice versa? Even if the pertinent local governments somehow agree upon one form of integration/annexation, running school districts, as well as public facilities like fire stations, community centers, and libraries, will be a major challenge as securing and managing the resources to operate them will bring additional administrative and financial burdens.
In fact, integrating or annexing administrative districts in Korea is rather rare. There have been several attempts to integrate or annex local governments, but the sheer number is small, and examples like the integration of Changwon, Masan, and Jinhae into the unified Changwon City, and the integration of Cheongju City and Cheongwon County into the unified Cheongju City, are cases where entire local government units are merged or annexed (Source: Yonhap News, 2024). Integrating Wirye is a completely different story and annexation in such circumstances is unprecedented.
Low Fiscal Autonomy of Local Governments
One of the main reasons these problems persist in Korea is that local governments cannot be financially independent. While laws allow local governments to adjust the standard tax rate through a flexible tax rate system, it is rarely used in practice, and most local governments apply the tax rate set by the central government considering the ‘equity of tax system' (Jeong, 2021). In other words, governments only exercise the legal authority to adjust tax rates when it does not bring any particular benefit or disadvantage. Furthermore, they have little, if not zero, authority to determine the tax base, another facet of tax revenues. The national government distributes subsidies to fill the discrepancy between fiscal needs and revenue. However, the decision is ultimately made by the national government. Local governments do not have the power to determine their revenues and they can hardly come up with a long-term policy.
The United States presents a contrasting case. While the federal government holds the highest authority, each state has discretion to determine the types of taxes and tax rates. For example, while Texas, where Shin-Soo Choo played, has no individual income tax, California has one of the highest, if not the highest, individual income tax rates. Delaware, Montana, and Oregon, for instance, have no sales tax. Furthermore, local governments—cities, towns, etc.—in New York State assess real estate and impose different tax rates. New York City prohibits right turns on red whereas it is legal to make a right turn on a red light as long as one yields to oncoming traffic first. Of course, this does not mean that state and local governments are completely fiscally independent. The U.S. also has a federal or state government system of tax collection and distribution through grants and subsidies, but local governments have higher fiscal autonomy compared to Korea.
The Need for Research on Tax Competition
Let's return to the case of Wirye. If Songpa District, Seongnam City, and Hanam City had not just waited for support from the national government but had ways to secure their own resources, they could have solved the problem of the delayed construction of the LRT project. The reality that the construction has been delayed for 16 years, despite residents paying additional contributions, clearly shows how important the fiscal autonomy of local governments is (Source: Chosun Biz, 2024).
However, if local governments' taxing powers were to increase in Korea, tax competition between local governments is inevitable. When local governments can autonomously adjust tax rates, they will inevitably compete to attract resources with minimal resistance from citizens. In other words, granting more taxing power to local governments can present new challenges as it provides local governments more autonomy. Ultimately, interactions between local governments, especially tax competition, play an important role, and the impact of such competition on the regional economy may be unneglectable.
Research Question
This study aims to examine the tax competition that arises in regions where tax jurisdictions are not completely independent from one another. Particularly in situations like Wirye, where multiple administrative districts overlap, the study will use a game-theoretic approach to model how local governments determine and interact with their tax policies, and to analyze the characteristics and results of the competition.
Specifically, this study aims to address the following key questions:
- What strategic choices do local governments make in overlapping tax jurisdictions?
- How is tax competition in this environment different from traditional models?
- What are the characteristics of the equilibrium state resulting from this competition?
- What are the impacts of this competition on the welfare of local residents and the provision of public services?
Through this analysis, the study aims to contribute to the effective formulation of fiscal policies in regions with complex administrative structures. It also expects to provide theoretical insights into the problems that arise in cases like Wirye, which can help inform policy decisions in similar situations in the future. The next chapter will go into further detail on tax competition and explain the specific models and assumptions used in this research.
Literature on Tax Competition
Tax competition refers to the competition between local governments to determine tax rates in order to attract businesses and residents. This can have a significant impact on the fiscal situation of local governments and the provision of public services.
The origin of the theory of tax competition can be traced back to Tiebout's (1956) "Voting with Feet" model. Tiebout argued that residents move to areas that provide the combination of taxes and public services that best matches their preferences. Later, Oates (1972) argued that an efficiently decentralized fiscal system can provide public goods, establishing this as the decentralization theorem.
However, Wilson (1986) and Zodrow and Mieszkowski (1986) pointed out that tax competition between local governments can lead to the under-provision of public goods. They argued that as capital mobility increases, local governments tend to lower tax rates, which can ultimately lead to a decline in the quality of public services.
Tax competition takes on an even more complex form in regions with overlapping tax jurisdictions. Keen and Kotsogiannis (2002) analyzed a situation where vertical tax competition, tax competition between different levels of governments such as those between central and local governments, and horizontal tax competition, tax competition between same level governments like those between local governments, occur simultaneously. They showed that in such an overlapping structure, excessive taxation can occur.
In the case of Wirye, the overlapping jurisdictions of multiple local governments make the dynamics of tax competition even more complex. Each government tries to maximize its own tax revenue, but at the same time, they must also consider the competition with other governments. This can lead to results that differ from traditional tax competition models.
In summary, the negative aspects of tax competition are:
- Decrease in tax revenue: Long-term shortage of tax revenue due to tax rate reductions
- Deterioration of public service quality: Reduction of services due to budget shortages
- Regional imbalances: Uneven distribution of public services due to fiscal disparities
- Excessive fiscal expenditures: Financial burden from various incentives to attract businesses
However, tax competition is not always negative. Brennan and Buchanan (1980) argued that tax competition can play a positive role in restraining the excessive expansion of government.
Research on tax competition in regions with overlapping tax jurisdictions is still limited. This study aims to analyze the dynamics of tax competition in such situations using a game-theoretic approach. This can contribute to the formulation of effective fiscal policies in regions with complex administrative structures.
Model
The Need for a Toy Model
It is extremely difficult to create a model that considers all the detailed aspects of the complex administrative system described earlier. In such cases, an effective approach is to create a toy model that removes complex details and represents only the core elements of the actual system. For example, to understand the principles of how a car moves, looking at the entire engine would be complicated, as it involves various elements like the fuel used, fuel injection method, cylinder arrangement, etc. However, through a toy model like a bumper car, one can, with relative ease, learn the basic principle that pressing the accelerator makes the car move forward, and pressing the brake makes it stop.
Similarly, in this study, we plan to use a toy model that removes complex elements in order to first understand the core mechanisms of tax rate competition. After understanding the core mechanisms through this study, the goal is to gradually add more complex elements to create a model that is closer to the actual system.
Assumptions
In game theory, a "game" refers to a situation where multiple players choose their own strategies and interact with each other. Each player tries to choose one or more optimal strategies to achieve their own goals, considering other players’ strategies.
In this study, we constructed a game by adding one overlapping region to the toy model of Itaya et al. (2008) and Ogawa and Wang (2016). Based on the Solow Model, a Nobel prize winning economic model for explaining long-term economic growth, the two models mainly investigated the capital tax rate competition between two regional governments within a country.
Specifically, there is a hypothetical country divided into three regions: two independent regions with asymmetric production technologies and capital factors, S and L, and a third region, O, which overlaps with the other two. The three regions have the independent authority to impose capital taxes with tax rates $\taui$for $\text{region}i$. The non-overlapping parts of S and L are defined as SS (Sub-S) and SL (Sub-L), respectively, and the overlapping regions between S and O, and L and O, are denoted as OS and OL, respectively (refer to Figure 2). S and L are higher level of jurisdictions that provide generic public good $G$while O is a special-purpose jurisdiction that provides a specific public good $H$tied to O.
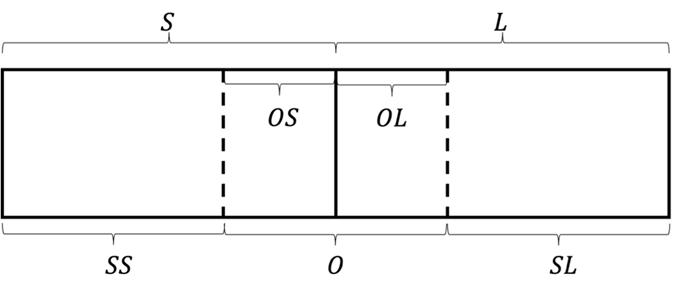
To intuitively observe only the "effect of the capital tax rate" due to the existence of overlapping regions, it is assumed that the population of regions S and L is the same. All residents in the country have the same preference and are inelastically supplying one unit of labor to companies in each region. This is a strong assumption because, under any circumstances, residents do not move and continue to work for their current company. However, it was a necessary assumption to simplify the game. Furthermore, it is assumed that companies employing residents in each region produce homogeneous consumer goods.
As mentioned above, S and L are assumed to have different capital factors and production technologies. Expressing this in a formula, the average capital endowment per person for the entire country is $\bar{k}$, and the average capital endowment per person for regions S and L are as follows:
[ \overline{ks}\equiv\bar{k}-\varepsilon, \qquad \overline{kL}\equiv\bar{k}+\varepsilon \qquad where\ \varepsilon\in\left(0,\ \bar{k}\right]\ \ and\ \ \bar{k}\equiv\ \frac{\overline{ks}+\overline{kL}}{2} ]
Even though the capital endowment may differ, capital can move freely. In other words, when a resident of region S invests capital in L, the cost is no different than investing capital in S.
To briefly introduce a few more necessary variables, the amount of capital required in region $i$ is denoted $Ki$, the amount of labor supplied is $Li$, and the labor and capital productivity coefficients are defined as $Ai$and $Bi > 2Ki$, respectively. Although regions S and L differ in capital production technology, there is no difference in labor production technology, so $AL = AS$while $BL \neq BS$. Region O does not occupy new territory in the hypothetical country but overlaps S and L regions in equal proportions in terms of area and population. Therefore, $AO = AL = AS$, and $BO$ would be the weighted average of $BL$ and $B_S$, with the weights being the proportion of capital invested in the OL and OS regions.
Market Equilibrium
Utilizing the variables introduced above, the production function under constant returns to scale (CRS) for region $i$used in this study can be expressed as follows.
[ Fi\left(Li,\ Ki\right)=AiLi+BiKi-\frac{Ki^2}{L_i} ]
Based on this production function, it is assumed that firms maximize their profits, and market equilibrium is assumed to occur when the total capital endowment and capital investment demand are equal. Based on this, we can calculate the capital demand and interest rates at market equilibrium:
[\begin{align}
r^ &= \frac{1}{2}\left(\left(BS+BL\right)-\left(\tauS+\tauL+\left(2-\alphaS-\alphaL\right)\tauO\right)\right)-2\bar{k} \
KS^* &= l\left(\bar{k}+\frac{1}{4}\left(\left(\tauL-\tauS-\left(\alphaL-\alphaS\right)\tauO\right)-\left(BL-BS\right)\right)\right) \
KL^* &= l\left(\bar{k}+\frac{1}{4}\left(\left(\tauS-\tauL+\left(\alphaL-\alphaS\right)\tauO\right)+\left(BL-BS\right)\right)\right) \
K{SS}^* &= \frac{2l}{3}\left(\bar{k}+\frac{1}{4}\left(\left(\tauL-\tauS+\left(2-\alphaL-\alphaS\right)\tauO\right)-\left(BL-BS\right)\right)\right) \
K{SL}^* &= \frac{2l}{3}\left(\bar{k}+\frac{1}{4}\left(\left(\tauS-\tauL+\left(2-\alphaL-\alphaS\right)\tauO\right)+\left(BL-BS\right)\right)\right) \
KO^* &= \frac{2l}{3}\left(\bar{k}-\frac{1}{2}\left(2-\alphaL-\alphaS\right)\tau_O\right)
\end{align*}
]
Here, $K{SS}=\alphaS KS$, $K{SL}=\alphaL KL$ while $0<\alphaS, \alphaL < 1$. Furthermore, we define $\theta$ as equal to $BL-BS$.
Residents maximize their post-tax income by investing capital, earning income from the market equilibrium return on capital $r^{\ast}$, and consuming it all. It is assumed that each tax jurisdiction provides public goods through taxes, and selects an optimal capital tax rate $\tau_i^{\ast}$to maximize the social welfare function, which is represented as the sum of individual consumption and the provision of public goods.
$u \left( Ci, Gi, Hi \right) \equiv Ci + Gi + Hi = $
[\begin{cases}
l\left( wi^* + r^* \bar{k}i \right) + Ki^* \taui + (1 - \alphai) Ki^* \tauO, & \text{for } i \in {S, L} \
\dfrac{2l}{3}\left( wO^* + r^* \bar{k} \right) + KO^* \tauO + (1 - \alphaS) KS^* \tauS + (1 - \alphaL) KL^* \tauL, \text{for } i = O
\end{cases}]
Then, tax rates at the market equilibrium are:
[\begin{align}
\tau_S^\ast &= \frac{4\varepsilon}{3}-\frac{\theta}{3}+\frac{\tau_L}{3}-\frac{2-3\alpha_S+\alpha_L}{3}\tau_O \
\tau_L^\ast &= -\frac{4\varepsilon}{3}+\frac{\theta}{3}+\frac{\tau_S}{3}-\frac{2-3\alpha_L+\alpha_S}{3}\tau_O \
\tau_O^\ast &= \frac{3\left(\alpha_L+\alpha_S\right)-4}{\left(2-\left(\alpha_L+\alpha_S\right)\right)\left(\alpha_L+\alpha_S\right)}\bar{k}=\Gamma\bar{k}
\end{align}]
Nash Equilibrium and Simulations
Nash Equilibrium
First, to briefly explain the Nash equilibrium, it refers to a state in which every player in a game has made their best possible choice and has no incentive to change their strategy any further. In other words, in a Nash equilibrium, no player can improve their outcome by changing their strategy, so all players continue to maintain their current strategies.
The tax rate at market equilibrium calculated in the previous section can be expressed as the optimal response function for each region. This is because, given the strategies of other regions, each region aims to maximize the social welfare function with its optimal strategy. In other words, this function shows which tax rate is most advantageous for region $i$ when considering the tax rates of other regions. Therefore, the Nash equilibrium tax rate can be derived based on the market equilibrium tax rate, and it can be expressed in the following formula.
[\begin{align}
\tau_S^N &= \varepsilon-\frac{\theta}{4}-\Gamma\left(1-\alpha_S\right)\bar{k} \
\tau_L^N &= -\left(\varepsilon+\frac{\theta}{4}\right)-\Gamma\left(1-\alpha_L\right)\bar{k} \
\tau_O^N &= \Gamma\bar{k}
\end{align}]
Furthermore, we can derive the following lemma and proposition.
Lemma 1. The sign of $\Phi\equiv\varepsilon-\frac{\theta}{4}$determines the net capital position of S and L, where L is the net capital exporter when it is a positive sign and vice versa.
Proposition 1. The sign of $\Gamma\equiv\frac{3\left(\alphaL+\alphaS\right)-4}{\left(2-\left(\alphaL+\alphaS\right)\right)\left(\alphaL+\alphaS\right)}$determines the effective tax rate of O and $\alphaL + \alphaS$ must be greater than 4/3 for O to provide a positive sum of special public good $H$.
Additionally, the capital demand and interest rates at the Nash equilibrium are as follows.
[\begin{align}
r^N&=\frac{1}{2\left(B_S+B_L\right)}-2\bar{k} \
K_S^N&=l\left({\bar{k}}_S+\frac{1}{2}\left(\varepsilon-\frac{\theta}{4}\right)\right) \
K_L^N&=l\left({\bar{k}}_L-\frac{1}{2}\left(\varepsilon-\frac{\theta}{4}\right)\right) \
K_{SS}^N&=\frac{2l}{3}\left({\bar{k}}_S+\frac{1}{2}\bar{k}\Gamma\left(1-\alpha_S\right)-\frac{1}{2}\left(\varepsilon+\frac{\theta}{4}\right)\right) \
K_{SL}^N&=\frac{2l}{3}\left({\bar{k}}_L+\frac{1}{2}\bar{k}\Gamma\left(1-\alpha_L\right)+\frac{1}{2}\left(\varepsilon+\frac{\theta}{4}\right)\right) \
K_O^N&=\frac{l}{3}\cdot\frac{4-\alpha_L-\alpha_S }{\alpha_L+\alpha_S}\bar{k}
\end{align}]
Simulations and Results
Since the Nash equilibrium is nonlinear, the results can be visualized through simulations. By adjusting $\alphaS$, $\alphaL$, $\epsilon$, and $\theta$ in the simulation, as mentioned in Lemma 1, one can see that when $\Phi$is greater than 0, the capital demand in region L decreases, and the capital demand in region S increases, leading L to export capital and S to import it (see Figure 3).

Based on the Nash equilibrium results, the utility that the representative residents of each region derive from public goods can be summarized as follows:
[up(Gi, Hi) =
\begin{cases}
\frac{KS^N \tauS}{l} & \text{for } i = SS \
\frac{KL^N \tauL}{l} & \text{for } i = SL \
\frac{KS^N \tauS}{l} + \frac{3 KO^* \tauO }{2l} & \text{for } i = OS \
\frac{KL^N \tauL}{l} + \frac{3 KO^* \tau_O }{2l} & \text{for } i = OL
\end{cases}]
This utility function can be visualized as is represented in Figure 4.
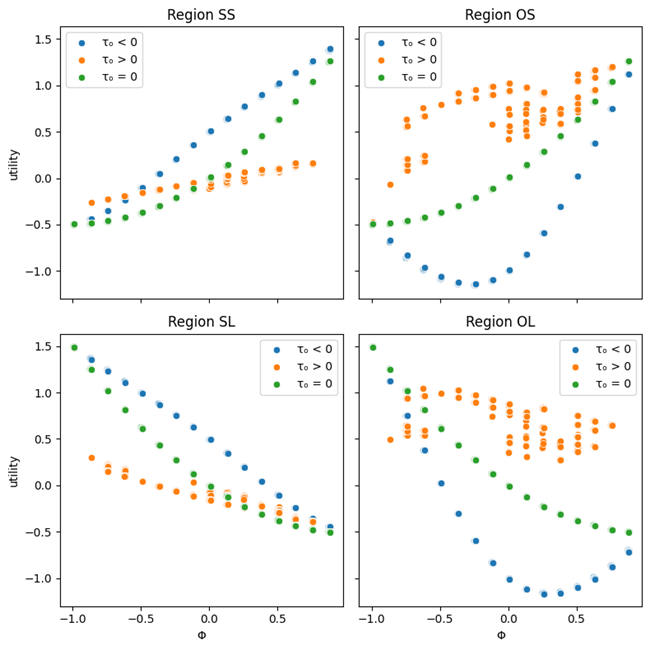
To summarize, in the case of region O, it is not directly affected by the tax rates of S and L, but it is directly influenced by the ratio of the allocated resources, i.e., the sum of $\alphaL$and $\alphaS$. S and L experience changes in their tax rates by $\Gamma\left(1-\alphaS\right)\bar{k}$ and $\Gamma\left(1-\alphaL\right)\bar{k}$, respectively, due to the existence of a special-purpose jurisdiction called O, compared to the scenario where such jurisdiction does not exist. Additionally, the net capital position is determined independently of the special-purpose jurisdiction.
Conclusion
The study examined how tax competition unfolds in regions with overlapping tax jurisdictions leveraging ideas from game theory. Specifically, a simplified toy model was constructed to understand the impact of tax competition in complex administrative structures, and from this, a Nash equilibrium was derived. Through this, it was identified that in the presence of overlapping tax jurisdictions, the patterns and outcomes of tax competition differ in certain ways from what is predicted in the models of Itaya et al. (2008) and Ogawa and Wang (2016).
However, this study has several limitations. First, the use of a toy model simplifies the complex nuances of real-world scenarios, meaning it does not fully reflect the various factors that could occur in practice. For instance, factors such as population mobility, governmental policy responses, various tax bases, and income disparities among residents were excluded from the model, which limits the generalizability of the results. Second, the economic variables assumed in the model, such as differences in capital endowments, production technologies, and resident preferences for public goods, may differ from reality, necessitating a cautious approach when applying these findings to real-world situations.
Despite these limitations, this study provides an important theoretical foundation for understanding the dynamics of tax competition in regions with overlapping administrative structures. Specifically, it suggests the need for a policy approach that considers the interaction between capital mobility and public goods provision, rather than merely focusing on a “race to the bottom” in tax rates. Future research should expand the model used in this study to include additional variables such as population mobility and governmental policy responses. Moreover, it will be essential to examine how tax competition evolves in repeated games. Finally, testing the model under various economic and social conditions will be crucial to improving its reliability for practical policy applications. By doing so, we can more accurately assess the real-world impacts of tax competition in complex administrative structures and contribute to designing effective policies.
Reference
Brennan, G., & Buchanan, J. M. (1980). The power to tax: Analytical foundations of a fiscal constitution. Cambridge University Press.
Keen, M., & Kotsogiannis, C. (2002). Does federalism lead to excessively high taxes? American Economic Review, 92(1), 363-370.
Itaya, J.-i., Okamura, M., & Yamaguchi, C. (2008). Are regional asymmetries detrimental to tax coordination in a repeated game setting? Journal of Public Economics, 92(12), 2403–2411.
Jeong, J. (2021). A Study on the Improvement of the Flexible Tax Rate System. Korea Institute of Local Finance.
Oates, W. E. (1972). Fiscal federalism. Harcourt Brace Jovanovich.
Ogawa, H., & Wang, W. (2016). Asymmetric tax competition and fiscal equalization in a repeated game setting. International Review of Economics & Finance, 41, 1–10.
Tiebout, C. M. (1956). A pure theory of local expenditures. Journal of Political Economy, 64(5), 416-424.
Wilson, J. D. (1986). A theory of interregional tax competition. Journal of Urban Economics, 19(3), 296-315.
Zodrow, G. R., & Mieszkowski, P. (1986). Pigou, Tiebout, property taxation, and the underprovision of local public goods. Journal of Urban Economics, 19(3), 356-370.